This:

In Thanksgiving Message, Trump Says ‘We’re Like a Third-World Country’

Yes, we are.

And what a lovely Thanksgiving message. We deserve it.

I received a number of comments from my previous post on MMT. No one gave me a satisfactory answer, but one commenter (Sam Levey) did actually answer the question.

Recall that I wanted to know what would have happened in 1998, when T-bill yields were 5%, if the Fed had suddenly doubled the base from $500 billion to $1000 billion by purchasing bonds. The standard model says that money is neutral in the long run, but the MMT textbook suggests that OMOs are “irrelevant”. But why?

Levey said:

MMTers essentially argue that any effect of OMOs have to happen through prices, not quantities. I.e. if it doesn’t affect interest rates, then it doesn’t affect inflation. And even if it does affect interest rates, it may not actually affect inflation, if there isn’t a large enough reaction from aggregate demand to actually cause prices to move.

That puzzled me for two reasons:

1. We know that banks don’t want to hold lots of excess reserves when interest rates are 5%, and the public’s demand for cash is very modest with 5% interest rates, say around 5% to 10% of GDP. So if interest rates don’t change, why would the public plus banks double their holdings of base money as a share of GDP? Why hold all this new zero interest base money? What about the hot potato effect?

2. If interest rate do adjust (and Levey implicitly allows for this option), then it is indeed possible that the public would hold the extra base money and prices and output would not change. So I’m going to assume that’s the assumption that MMTers would make. Option #1 is too bizarre to contemplate. After all, how plausible is it that the Fed could dump another $500 billion in base money into an economy with T-bill yields of 5%, doubling the monetary base, without dramatically reducing interest rates? (Sure, John Cochrane might argue that rates would go up due to the Fisher effect, but in that case MMTers would be wrong in claiming no effect on inflation.)

And if interest rates do fall to zero, bringing mortgage rates from say 7% to 2% in a booming economy . . . forever . . . how likely is it that this action is “irrelevant” for the broader economy? You wake up in the summer of 1998 and read the Fed cut rates from 5% to 0%—seriously; how do you react? Irrelevant???? Yeah, savers earn less interest—but irrelevant for investment decisions like building a new house?

I say “forever” because if you argue the interest rate reduction is just temporary then there would be a long run inflation effect from people trying to get rid of excess cash balances once interest rates rose again. That’s actually what would occur, but they seem to deny it. So the irrelevance claim seems to require that interest rates fall permanently.

I just don’t get it. What’s the new long run equilibrium for base money demand, interest rates, the price level, etc. after a big increase in the base when nominal interest rates are strongly positive?

I don’t know if Sam Levey correctly characterized MMT theory, but this explanation doesn’t really provide a satisfactory answer to my question. I wish MMTers would say, “I see why you are puzzled, but here’s the intuition of why you are wrong”. But some of the responders didn’t seem to understand why this claim is so perplexing at first glance. People, you need to understand the alternative model. Don’t be like those Trumpistas that can’t figure out why most of us are skeptical of claims of massive election fraud.

If I were to try to develop a radical new macro theory, I’d try to come up with a way of explaining my new model using the framework of existing models. Actually, I often do that here, translating market monetarism into New Keynesian language. I’m not seeing that with MMT. And it’s not just me. I see other bloggers like Noah Smith, Paul Krugman, Brad DeLong, Nick Rowe, etc., who seem to have an equally hard time trying to figure out what MMTers are claiming. MMTers should understand why we are confused, and have plausible answers. One sign that you are truly on top of an issue is if you can see why others hold a different view, and explain things in their language.

PS. And please don’t tell me the Fed can’t increase the base because they target interest rates. That’s completely irrelevant to the question at hand. It’s a thought experiment.

Update: After I wrote this I saw a few more responses. Sam Levey’s second response was more exasperating:

“This is one of those ‘paradigm shift’ issues. Your language doesn’t work within our paradigm, and clearly ours doesn’t work within yours. In our a paradigm “what are the effects of OMOs” isn’t a sensible question, because OMOs are not a discretionary instrument.”

So the MMT textbook says OMOs are irrelevant. When I call them on this point, asking what would happen if the Fed had bought $500 billion in bonds in 1998, they retreat to the claim that discretionary OMOs are impossible. Then why didn’t the textbook just say so! That’s a radically different claim, not to mention a false claim; discretionary OMOs are possible as long as you are willing to allow interest rates to move, which is exactly the monetarist position.

I see why Paul Krugman called debating MMTers a game of Calvinball.

And this is far worse (my statement then his response):

“Nominal lending is reserve constrained and real lending is demand constrained.”

I honestly have no idea what this means. “Nominal lending” and “real lending” refer to the exact same thing, but measured in different units. How can one of them be reserve-constrained and the other not be?

This is why I suspect that MMTers do not understand the theories they are criticizing. You may disagree with me on reserves and lending, but how can one fail to understand a basic EC101 point about money neutrality, about the distinction between what determines real variables and what determines nominal variables?

As for his question, take a look at figures for nominal and real lending in Zimbabwe in 2008. Nominal lending went up perhaps a trillion fold, while real lending probably declined.

I have a new Mercatus working paper explaining why monetary economics needs to stop focusing on interest rates. Of all the economics papers that I have written, this one best captures how my view of monetary economics differs from the mainstream. Here is the abstract:

In recent years, Keynesians and NeoFisherians have debated whether a low-interest-rate policy is inflationary or disinflationary. Both sides are wrong; interest rates are not a useful indicator of the stance of monetary policy. Some contractionary monetary policies lead to lower interest rates, while other contractionary monetary policies lead to higher interest rates. Instead, economists should use market expectations of inflation, nominal GDP growth, or both to measure the stance of monetary policy. Furthermore, the Fed should no longer target interest rates.

There’s no math because all I really do is use off the shelf concepts like the interest parity condition, Dornbusch overshooting, and the Fisher effect to look at interest rates from a different perspective. I’m not trying to re-invent the wheel, just show that the wheel needs to be re-aligned.

Most economists will probably hate this paper. But if there are a few younger readers that get something out of it, I’ll be happy. Maybe one of them will rewrite the paper using math.

BTW, John Cochrane has a new post that reconciles the Keynesian and NeoFisherian views of interest rates. He suggests that perhaps higher interest rates are disinflationary in the short run and inflationary in the long run—basically Milton Friedman’s view. Here’s Cochrane:

Reconciliation: 

In sum, once we include a multitude of plausible fractions that send inflation temporarily the other way, including the long-term bond effect and household financial frictions, a negative response to temporary interest rate rise like Sweden is consistent with a neo-Fisherian prediction that in the very long run higher interest rates produce higher inflation, and thus also consistent with the lack of a spiral at the zero bound.

But I move somewhat in Lars’ [Svensson] direction. Just how relevant is this observation to policy? When the central bank can move interest rates, it may well want to push rates around by exploiting the temporary negative sign.  “Temporary” can be a long time.  Even if the long-run effect is positive, the central bank may move inflation up more quickly by lowering rates, pushing inflation up with the short-run negative effect, and then then quickly getting on top of inflation. Which is just what central banks classically do, and exactly what they do if the economy is unstable as well. They may never notice the positive possibility, and may never have the patience to wait for it. Thus, the neo-Fisherian possibility may be completely irrelevant in normal times. 

But when the central bank cannot lower interest rates, then the slow, preannounced, persistent, we-wont-give-up, and whatever else needed to overcome or wait out temporary forces in the other direction, may still be a useful policy for liftoff. One might indeed read the US interest rate increases — known years in advance — in that light. 

That’s plausible, but it’s not my reconciliation. I believe that monetary stimulus can either raise or lower interest rates even in the short run, as the following two exchange rate graphs illustrate:

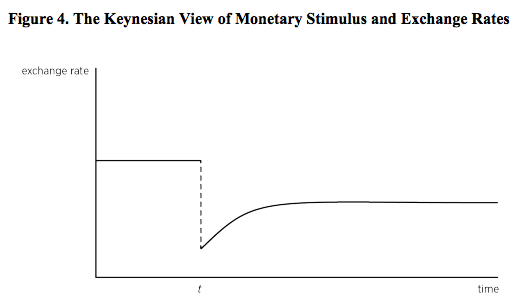
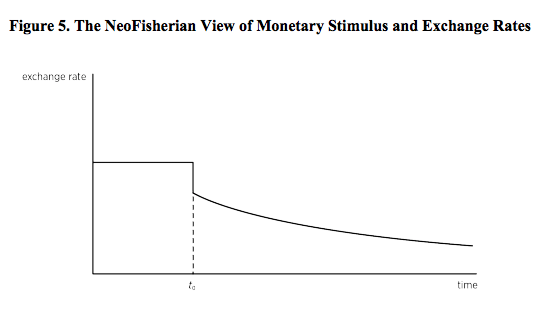
PPS. I said most economists will hate my paper. Nick Rowe is one possible exception. Here he comments on Cochrane’s post:

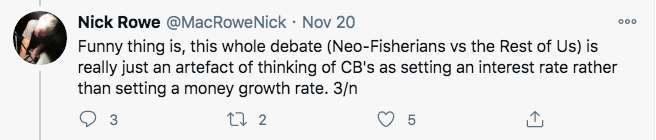
Or setting the exchange rate growth rate. Or setting the TIPS spread. Or setting the NGDP futures price growth rate.

Please, anything but interest rates.

From Yahoo.com:

Modern Monetary Theory is a term that one encounters with increasing frequency. It is often applied to a specific policy, such as advocacy of expansionary fiscal policy. But that’s not a very useful definition. Lots of economists now advocate expansionary fiscal policy in the current environment of very low interest rates and high unemployment.

MMT is more than fiscal stimulus; it is a model of the macroeconomy. In order to better understand the MMT model I’ve been reading “Macroeconomics”, an undergraduate textbook written by William Mitchell, Randall Wray and Martin Watts. While MMT is not my cup of tea, I don’t want to be unfair in my appraisal. Thus I’ll discuss one potential problem here (and another today over at Econlog), and try to elicit feedback from MMTers—what am I getting wrong? Am I being unfair? If so, what’s the intuition that I’m missing?

On page 342 they make an assertion that caught my attention:

Monetarists are hostile to the creation of base money to finance deficits because they claim it is inflationary due to the Quantity Theory of Money (QTM). MMT advocates would first highlight institutional practice, namely that net treasury spending initially causes an equal increase in base money.

Second, they would challenge the theory of inflation based on QTM, and argue that if a fiscal deficit gives rise to demand pull inflation, then the ex post composition of  ΔB +  ΔMb in Equation (21.1) is irrelevant. Overall spending in the economy is the driver of the inflation process, and not the ex post distribution of net financial assets created between bonds and base money.

A few initial observations:

1. The first paragraph seems misleading, as it may give students the false impression that the Fed does not determine the stock of base money. But I’d like to focus on the second paragraph.

2. The second paragraph seems to apply to all open market purchases, not just those that occur when market interest rates equal the interest rate on excess reserves (IOER). That’s clear from the rest of the book, which focuses heavily on historical examples from the 1960s, 1970s and 1980s. So for the rest of the post I’ll consider OMOs that occur in a world where nominal interest rates are positive and there is no IOER, i.e. the pre-2008 world. To be sure, I don’t think their claim is even true in a world with IOER, but it’s at least more defensible in today’s world.

3. Most mainstream economists (including John Maynard Keynes) would not agree with the claim that a purchase of interest-bearing bonds with zero interest base money is “irrelevant”.

4. I’m focusing on this point because much of the analysis in this textbook hinges on this claim. If it’s false (and I think it is) then the rest of the book sort of falls apart. For instance, the book assumes that demand management is done by the fiscal authority, whereas in the real world central banks determine aggregate demand (at least when interest rates are positive).

OK, so why do MMTers believe that OMOs don’t matter? Suppose we go back to the economy of the 1990s, when risk-free interest rates were often about 5%. Assume the monetary base is $500 billion (as in 1998). Now consider this thought experiment. The Fed buys another $500 billion in Treasury bonds, paid for with zero interest base money. Is that action actually “irrelevant” because the sum of base money and publicly held debt doesn’t change?

Both monetarists and Keynesians would predict that this action would boost output in the short run, and cause both P and NGDP to double in the long run. Monetarists would point to the creation of huge excess cash balances. Banks would try to get rid of their excess reserves by purchasing assets. Over time, some of the excess reserves would leak out as cash in circulation, but this would cause excess cash balances for the public. Only when the price level doubled would the public and banks be willing to hold $1000 billion in base money, twice as much as before.

Now you might argue that this is the monetarist view, which has fallen out of favor. But Keynesian would make roughly the same prediction.

In the Keynesian model, the huge open market purchase would sharply depress interest rates, to a level far below the natural rate of interest. This would cause aggregate demand to rise sharply. Aggregate demand would continue to increase until the market interest rate was once again equal to the natural rate. But that could only occur when the price level had doubled. Even in the Keynesian model, one-time increases in the monetary base are neutral in the long run.

So what do MMTers think would happen? That’s what I can’t figure out. Here are some possibilities:

1. Maybe the MMT claim that the composition is “irrelevant” is a claim that these assets are close substitutes. Swapping cash for bonds doesn’t matter. Interest rates, output and inflation are not affected. But is this plausible? Why would the Fed’s decision to double the monetary base cause the public and banks to wish to hold twice as much zero interest base money as a share of GDP? For instance, if banks can earn 5% on T-bills and 0% on reserves, why would they choose to hold more excess reserves? Ditto for the public’s holding of cash.

2. Maybe the MMT claim is that the composition does affect interest rates, but not the broader economy. That is, maybe they are claiming that a big open market purchase would drive interest rates much lower, perhaps even to zero, without impacting output or inflation. But how plausible is the claim that in 1998 the Fed could have injected enough base money to drive rates to zero without triggering inflation?

NeoFisherians correctly point out that low interest rates are often associated with low inflation. But that’s probably due to reverse causation—the Fisher effect. I know of no case where a central bank injected massive quantities of reserves into an economy with market interest rates well above zero and with no IOER (i.e. an economy like the US in 1998) without triggering high inflation. And I know of many dozens of cases where such a policy did create high inflation.

How plausible does it seem that you could take an economy like the US in 1998, cut Treasury bill yields from 5% to 0% vie OMOs, which would dramatically reduce mortgage interest rates, and not trigger a sharp increase in aggregate demand? I just don’t get what the MMTers are claiming here. If interest rates didn’t fall then you get runaway inflation via the hot potato effect, and if interest rates immediately plunged to zero you’d get a big rise in AD because (unlike in cases like the deflationary 1930s or 1990s Japan) you’d be sharply depressing market interest rates to well below the natural rate for a healthy economy.

What do MMTers think would have happened if the Fed had bought $500 billion in bonds in 1998, and announced that the increase was permanent? And why?

Even if I am misinterpreting their claim and they respond, “Yes, MMTers admit that open market purchases are expansionary”, it would not make me think I’ve wasted my time with this post. That answer would imply that there are other major problems with the textbook, which is mostly written using the implicit assumption that monetary policy does not determine aggregate demand.

I’ll have many more questions on MMT.

PS. And can we count the %$*#@&$ votes! Isn’t that the point of elections?